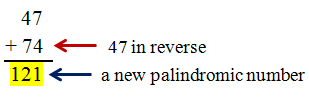I was getting ready to pay for my meal at a buffet when I noticed the cashier's name tag. It read "Anna" to which I replied, "Your name is a palindrome!" The cashier just stared at me in disbelief. I explained that a palindrome was letters that read the same backwards as forwards. Because you could read her name forwards and backwards, it qualified as a palindrome. She replied that she remembered a math teacher talking about those because of patterns (I love that math teacher), and she remembered the phrase "race car" was a palindrome. We then began sharing palindromes that we knew such as radar, level and madam while my family waited impatiently in line. (Sometimes they have little patience with my math conversations.)
The word palindrome is derived from the Greek word palíndromos, which means "running back again". A palindrome can be a word, phrase or sentence which reads the same in both directions such as: "Eva, can I stab bats in a cave?" or "Was it a car or a cat I saw?" or "Rats live on no evil star."
Suppose a person starts with the number one and lists the palindromic numbers in order: 11, 22, 33, 44, 55...etc. Can you continue the list?
Did you notice that palindromic numbers are symmetrical? Look carefully at the 17371 shown above. It is symmetrical (when a figure can be folded along a line so the two halves match perfectly) on either side of the three whether read left to right or vice versa.
Palindromic numbers are very simple to generate from other numbers with the help of addition.
Try this:
- Write down any number that has more than one digit. I will use 47.
- Write down that number in reverse beneath the first number. (See illustration below.)
- Add the two numbers together. (121)
- 4. The sum of 121 is undeniably a palindrome.
- Start with 75.
- Reverse 75 which makes 57.
- Add 75 and 57 and you get 132. The answer 132 is not a palindrome.
- SO reverse 132, and it becomes 231.
- Add 132 and 231, and the answer is 363.
- Since 363 is a palindrome, we are done!
- Begin with 255.
- Reverse 255 to get 552.
- Add 255 and 552. The answer is 807 which is not a palindrome.
- SO reverse 807 to get 708.
- Add 807 and 708. The answer of 5151 is not a palindrome.
- SO reverse 1515 to get 5151.
- Add 1515 and 5151 which is 6666.
- This is a palindrome; so, we are done!